Question

Hello, can you please help me solve question six !

Answer 1

QUESTION A

The equation is given to be:

`\cos x-\sec x=1`

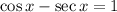
Step 1: Apply the identity:

`\sec x=(1)/(\cos x)`

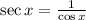
Therefore, we have:

`\cos x-(1)/(\cos x)=1`

Step 2: Multiply all through by cos x:

`(\cos x)^2-1=\cos x`

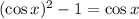
Step 3: Rewrite the equation

`(\cos x)^2-\cos x-1=0`

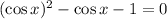
Step 4: Make the substitution for cos x = m

`\begin{gathered} \therefore \\ m^2-m-1=0 \end{gathered}`

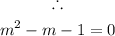
Step 5: Solve the quadratic equation using the quadratic formula

`\begin{gathered} m=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ a=1,b=-1,c=-1 \\ m=\frac{-(-1)\pm\sqrt[]{(-1)^2-4*1*(-1)}}{2*1} \\ m=\frac{1\pm\sqrt[]{1+4}}{2} \\ m=\frac{1\pm\sqrt[]{5}}{2} \\ \therefore \\ m=\frac{1+\sqrt[]{5}}{2},\frac{1-\sqrt[]{5}}{2} \end{gathered}`

Step 6: Substitute for m back into the solution of the quadratic equation

`\begin{gathered} \cos x=\frac{1+\sqrt[]{5}}{2} \\ or \\ \cos x=\frac{1-\sqrt[]{5}}{2} \end{gathered}`

Step 7: Solve for x by finding the cosine inverse of the solutions

or

`x=\cos ^(-1)(\frac{1-\sqrt[]{5}}{2})=128.17`

The value of x is 128.17.

QUESTION B

The equation is:

`\cos x+\sec x=1`

Step 1: Rewrite the equation

`\cos x+(1)/(\cos x)=1`

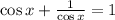
Step 2: Multiply all through by cos x

`(\cos x)^2+1=\cos x`

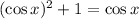
Step 3: Rearrange the equation terms

`(\cos x)^2-\cos x+1=0`

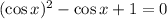
Step 4: Make the substitution for cos x = m

`m^2-m+1=0`

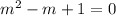
Step 5: Solve the quadratic equation

`m=\frac{1+i\sqrt[]{3}}{2},\frac{1-i\sqrt[]{3}}{2}`

Step 6: Substitute for m back into the solution of the quadratic equation

`\begin{gathered} \cos x=\frac{1+i\sqrt[]{3}}{2} \\ or \\ \cos x=\frac{1-i\sqrt[]{3}}{2} \end{gathered}`

Step 7: Solve for x by finding the cosine inverse of the solutions

There is no solution for x.