Answer with explanation:
Mean
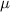 = 50
= 50
Standard Deviation
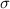 =8
=8

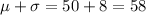

⇒When you will read the normal distribution curve, you will find that, about 68% of the data falls between 1 standard deviation right and 1 standard deviation left of the mean.
→68% of the data falls between 42 and 58.