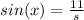Answer:
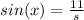
Explanation:
we know that
In a right triangle
the tangent of an angle is equal to divide the opposite side of the angle by the adjacent side of the angle
the function cosine of an angle is equal to divide the adjacent side of the angle by the hypotenuse
the function sine of an angle is equal to divide the opposite side of the angle by the hypotenuse
so
In this problem we have
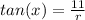
the opposite side angle x is equal to

the adjacent side angle x is equal to
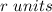
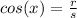
the adjacent side angle x is equal to
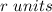
the hypotenuse is equal to
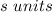
Hence