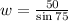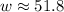Question

х W 50 75 Solve for: W = X= X= Round to the nearest tenth. Given that the triangle is Right-triangle.

Answer 1

To solve for X

X + 90 + 75 = 180° (Sum of interior angle of a triangle)

X + 165 = 180°

Subtract 165 from both-side of the equation

X = 180° - 165°

X = 15°

To solve for x

opposite =50

Adjacent = x

θ=75

Using the trigonometric ratio;

SOH CAH TOA

`\begin{gathered} \tan \theta=\frac{opposite}{\text{adjacent}} \\ \\ \tan 75=(50)/(x) \\ \\ x\tan 75=50 \\ \\ x=(50)/(\tan 75) \end{gathered}`
`x\approx13.4`

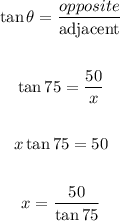
To solve for w,

opposite = 50

hypotenuse= w

θ=75

Hence, we will use sine.

`\sin \theta=\frac{\text{opposite}}{\text{hypotenuse}}`
`\sin 75=(50)/(w)`

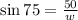
Cross-multiply

`w\sin 75=50`

Divide both-side by sin75

`w=(50)/(\sin 75)`
`w\approx51.8`