Question

The friends now consider a charged particle with charge q that moves in a region containing a uniform electric field, E, similar to what they've observed in the simulation. They let the length of the plates creating the uniform electric field be L, and the distance between the plates be d. Alston challenges Thana to calculate the maximum speed, v, the charged particle could be moving if it enters the space halfway between and parallel to the two plates and just barely manages to strike one of the plates. Which response is correct

Answer 1

Step-by-step explanation:

The charged particle will experience a force towards one of the plates , in direction perpendicular to its initial velocity because electric field is between the plates .

The force experienced by it = q E

acceleration a = qE / m , where m is mass of the charged particle .

The particle will have two velocity at the time it leaves the space between plates .

Horizontal velocity = initial horizontal velocity = v .

vertical velocity is created by force qE

Time taken by horizontal velocity to cover distance L

t = L / v

vertical velocity attained V = u + at

0 + at ( time taken by vertical velocity and time taken by horizontal velocity are equal )

vertical velocity = (qE/ m) x (L/v) = qEL / mv

Resultant velocity =
`\sqrt{((v)/(1) )^2+((qEL)/(mv))^2 }`

=
`\sqrt{(v^2+((qEL)/(mv))^2 }`

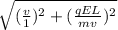
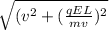
This gives the magnitude of resultant velocity .