Question

How many moles of ions are in 285 ml of 0.0150 m mgcl2?

Answer 1

MgCl₂)= Mg²⁺ + 2Cl⁻
V(MgCl₂)=285cm³=0,285dm³
c(MgCl₂)=0,015 mol/dm³
n(MgCl₂)=c·V= 0,015 mol/dm³ · 0,285dm³ = 0,0042 mol
n(Mg²⁺)=n(MgCl₂)=0,0042 mol
n(Cl⁻)=2n(MgCl₂)=0,0084 mol

Answer 2

Answer : The number of moles of
`Mg^(2+)` and
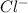`Cl^-` ions are 0.00428 and 0.00856 moles respectively.

Explanation :

Molarity : It is defined as the number of moles of solute present in one liter of volume of solution.

Formula used :

`\text{Molarity}=\frac{\text{Moles of solute}}{\text{Volume of solution (in L)}}`

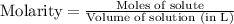
In this question, the solute is
`MgCl_2`.

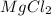Given :

Volume of solution = 285 mL = 0.285 L (1 L = 1000 mL)

Molarity = 0.0150 M

Now put all the given values in this formula, we get:

`0.0150M=\frac{\text{Moles of }MgCl_2}{0.285L}`

`\text{Moles of }MgCl_2=0.0150M* 0.285L`

`\text{Moles of }MgCl_2=0.00428moles`

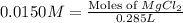
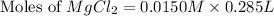
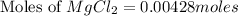
Thus, the number of moles of
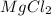`MgCl_2` are 0.00428 moles.

By the stoichiometry we can say that, 1 moles of
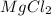`MgCl_2` dissociates to give 1 mole of
`Mg^(2+)` ion and 2 moles of
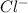`Cl^-` ions.

So,

Number of moles of
`Mg^(2+)` ion = 0.00428 mole

Number of moles of
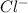`Cl^-` ion = 2 × 0.00428 = 0.00856 mole

Therefore, the number of moles of
`Mg^(2+)` and
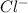`Cl^-` ions are 0.00428 and 0.00856 moles respectively.