Question

To three decimal places, find the value of the first positive x-intercept for the function f(x) = 2cos(x + 3).

2.712
−1.429
1.712
−1.712

Answer 1

it is definitely not b

Answer 2

The first positive x-intercept for the function
`f(x) = 2cos(x + 3)` is
`(1.712, 0)`.

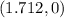Explanation:

The x-intercept is the point where a line crosses the x-axis,

To find the x-intercept for the function
`f(x) = 2cos(x + 3)`, let's substitute f(x) = 0 into the equation and solve for x:

`2\cos \left(x+3\right)=0\\\\(2\cos \left(x+3\right))/(2)=(0)/(2)\\\\\mathrm{General\:solutions\:for}\:\cos \left(x+3\right)=0\\\\x+3=(\pi )/(2)+2\pi n,\:x+3=(3\pi )/(2)+2\pi n`

We want the value of the first positive x-intercept so we take the value of
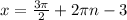`x=(3\pi )/(2)+2\pi n-3` when n = 0.

`x=(3\pi)/(2)+2\pi\cdot 0-3\\\\x=(3\pi )/(2)-3\approx 1.712`

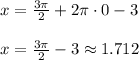
We can check our answer with the graph of the function. We can see that we get the same answer.