Question

1Jessie draws triangle ABC on a coordinate grid. The slope of line segment AB is \frac{3}{4} 43 . Jessie then transforms triangle ABC using a single transformation to create triangle A'B'C'. She claims the slope of A'B' will still be \frac{3}{4} 43 . For the transformation below, indicate whether it supports or does not support Jessie's claim.Rotation of 180o around the origin.

Answer 1

Step-by-step explanation

The slope of a line is a measure of its steepness. Mathematically, the slope is calculated as "rise over run" (change in y divided by change in x).

`\text{ Slope }=\frac{\text{ rise}}{\text{ run}}`

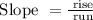
Since the slope of the line segment AB is 3/4, we know that its rise is 3, and its run is 4.

`\text{ Slope of line segment AB }=\frac{\text{ rise}}{\text{ run}}=(3)/(4)`

So, the coordinates of points A and B could be A(0,0) and B(4,3).

On the other hand, the rule for a rotation by 180° about the origin is:

`(x,y)\rightarrow(-x,-y)`

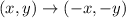
Then we can apply the above rule and calculate the coordinates of line segment A'B'.

Finally, let us find the slope of the line segment A'B'.

As we can see, the slope of the line segment A'B' is also 3/4.

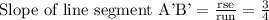
`\text{Slope of line segment A'B'}=\frac{\text{r\imaginaryI se}}{\text{run}}=(3)/(4)`Answer

Supports Jessie's claim