Question

The cost of 2 bottles of water and 4 apples is $5.50. The cost of 3 bottles of water and 5 apples is $7.50. Find the cost of one apple and the cost of one bottle of water using elimination.

Answer 1

You could use a simultaneous equation to work this out. The price of an apple would be 75 cents. The price of a bottle of water would be $1.25.

Answer 2

Apple: $0.75

Water bottle: $1.25

Explanation:

Let a represent cost of each apple and b represent cost of each water bottle.

We have been given that the cost of 2 bottles of water and 4 apples is $5.50. We can represent this information in an equation as:

`2b+4a=5.50...(1)`

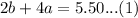
We are also told that the cost of 3 bottles of water and 5 apples is $7.50.We can represent this information in an equation as:

`3b+5a=7.50...(2)`

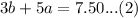
From equation (1), we will get:

`b=(5.50-4a)/(2)`

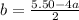
Upon substituting this value in equation (2), we will get:

`3((5.50-4a)/(2))+5a=7.50`

`(3\cdot 5.50-3\cdot 4a)/(2)+(10a)/(2)=7.50`

`(16.5-12a+10a)/(2)=7.50`

`(16.5-2a)/(2)\cdot 2=7.50}\cdot 2`

`16.5-2a=15`

`16.5-16.5-2a=15-16.5`

`-2a=-1.5`

`(-2a)/(-2)=(-1.5)/(-2)`

`a=0.75`

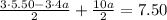
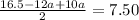
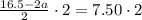
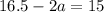
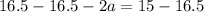
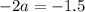
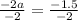
Therefore, cost of each apple is $0.75.

Upon substituting
`a=0.75` in equation
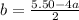`b=(5.50-4a)/(2)`, we will get:

`b=(5.50-4(0.75))/(2)`

`b=(5.50-3)/(2)`

`b=(2.50)/(2)`

`b=1.25`

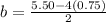
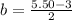
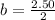
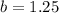
Therefore, cost of each bottle of water is $1.25.