Question

Given the regular hexagon, find the measure of each numbered angle.

Answer 1

I believe that the answer would be C. because each triangle that is “broken up” in the hexagon is equilateral meaning that angle 1 and angle 3 would be congruent and equal to 60, and since angle 2 is half the measure of angle 1 it would be 30. Hope this helps!

Answer 2

m∠1=60°, m∠2=30°, m∠3=60°

Option C is correct.

Explanation:

Given a regular hexagon

we have to find the measure of ∠1, ∠2 and ∠3

As the angle formed at the centre of circle is 360° and the diagonals of hexagon divides the hexagon into 6 congruent triangles implies the angle at the centre of these 6 congruent triangles are equal.

∴
`\angle 1 =(360)/(6)=60^(\circ)`

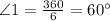
In ΔFOE, by angle sum property

∠FOE+∠OEF+∠3=180°

60°+2∠3=180° (∵ OF=OE both are radius)

2∠3=120°

∠3=60°

⇒ ΔFOE is equilateral triangle.

Hence, all 6 are equilateral triangles

In ΔOGD, by angle sum property

∠2+∠OGD+∠ODG=180°

∠2+90°+60°=180

∠2=30°

∠1=60°, ∠2=30°, ∠3=60°

Hence, option C is correct.