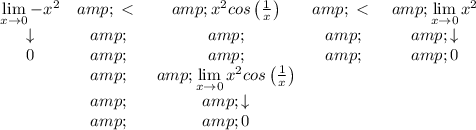Question

Could some explain the sandwich theorem (calculus/ limits)

Answer 1

`\bf \textit{we know the range for }cos\left( (1)/(x) \right)\textit{ is }[-1,1]\textit{ therefore} \\\\\\ -1~\ \textless \ ~cos\left( (1)/(x) \right)~\ \textless \ ~1\impliedby \textit{multiplying all sides by }x^2 \\\\\\ -1x^2~\ \textless \ ~x^2cos\left( (1)/(x) \right)~\ \textless \ ~1x^2\implies -x^2~\ \textless \ ~x^2cos\left( (1)/(x) \right)~\ \textless \ ~x^2`

if the limit of -x² goes to "something", and the limit of x² goes to the same "something", if their limit coincide, and yet they're bounding the cosine expression, therefore, since the cosine expression is "sandwiched" between -x² and x², then the cosine expression "squeezes in" that little sliver between both -x² and x², and will inevitably go to the same limit.


`\bf \begin{array}{ccccc} \lim\limits_(x\to 0) -x^2&\ \textless \ &x^2cos\left( (1)/(x) \right)&\ \textless \ &\lim\limits_(x\to 0) x^2\\ \downarrow &&&&\downarrow \\ 0&&&&0\\ &&\lim\limits_(x\to 0)x^2cos\left( (1)/(x) \right)\\ &&\downarrow \\ &&0 \end{array}`