Question

Complete the point-slope equation of the line through (1,0) and (6,-3).

Use Exact Numbers.

y-(-3)=_________

Answer 1

`y-(-3)=-(3)/(5)(x-6)`

Explanation:

If a line passes through two points
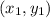`(x_1,y_1)` and
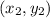`(x_2,y_2)`, then equation of line is

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

The point slope form of line is

`y-y_1=m(x-x_1)`

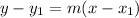
where, m is slope.

It is given that the line passes through the points (1,0) and (6,-3).

In the given equation y-(-3)=___,
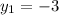`y_1=-3`. It means

`(x_1,y_1)=(6,-3)`

`(x_2,y_2)=(1,0)`

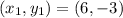
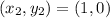
So, the point slope form of the given line is

`y-(-3)=(0-(-3))/(1-6)(x-6)`

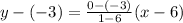
On simplification we get

`y-(-3)=-(3)/(5)(x-6)`

Therefore the point slope form of the given line is
`y-(-3)=-(3)/(5)(x-6)`.

Answer 2

(1,0),(6,-3)
slope(m) = (-3 - 0) / (6 - 1) = -3/5

y - y1 = m(x - x1)
slope(m) = -3/5
(6,-3)....x1 = 6 and y1 = -3
now sub....and pay attention to ur signs
y - (-3) = -3/5(x - 6)......this is not the final answer....but it seems to be what ur question is asking for.