Question

In this figure , what is angle ABE?What is angle DBE? enter your answer in the boxes

Answer 1

The angle ∠DBC is a right angle divided into two by line segment BE, the resulting angles ∠DBE and ∠EBC are corresponding angles, which means that they add up to 90º, so that:

`\angle\text{DBE}+\angle\text{EBC}=90º`

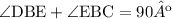
We know that ∠EBC=64.5º, so replace this measure in the expression above to determine the angle ∠DBE:

`\begin{gathered} \angle\text{DBE}+64.5=90 \\ \angle\text{DBE}=90-64.5 \\ \angle\text{DBE}=25.5º \end{gathered}`

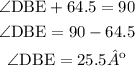
Now, ∠ABD and ∠DBC are supplementary, which means that they add up to 180º. We know that ∠DBC is a right angle, so if both angles are supplementary, then ∠ABD must be a right angle too.

Following the angle addition postulate, we can determine that the measure of ∠ABE is equal to the sum of the measures of the angles ∠ABD and ∠DBE, so that:

`\begin{gathered} \angle\text{ABE}=\angle\text{ABD}+\angle\text{DBE} \\ \angle\text{ABE}=90+25.5 \\ \angle\text{ABE}=115.5º \end{gathered}`

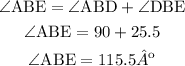
So angle ∠DBE= 25.5º and ∠ABE=115.5º