Question

4. To save for Malcom's education, his parents put $1,200 into a savings account whenMalcom was born in 2006. Every birthday, Malcolm's parents deposit 10% more than theprevious year.Part A: Determine the explicit formula to determine the amount of money deposited intoMalcolm's education fund. Assume ao = 2006Explicit Formula:Part B: Complete the table below.YearMoneyDeposited200612002010201420182022Part C: Determine the amount of money Malcom's parents contribute to the educationfund when Malcom graduates high school and furns 18.

Answer 1

To save for Malcom's education, his parents put $1,200 into a savings account when

Malcom was born in 2006. Every birthday, Malcolm's parents deposit 10% more than the

previous year.

Part A

In this problem we have an exponential function of the form

y=a(1+r)^x

where

a is the initial deposit

r is the rate of change

x is the number of years

y is the amount deposited in the saving account

so

we have

a=$1,200

r=10%=0.10

substitute

y=1,200(1+0.10)^x

y=1,200(1.10)^x --------> explicit formula

Part B

2006 -----> For x=0 -------> y=$1,200

2010 -----> For x=4 -----> y=$1,756.92

2014 ----> For x=8 -----> y=$2,572.31

2018 ----> For x=12 ----> y=$3,766.11

2022 ----> For x=16 ----> y=$5,513.97

Part C

we have an geometric sequence

To find out the sum

we use the formula

`S=(a(1-r^n))/(1-r)`

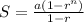
where

a is the first term

n is the number of terms

r is the common ratio

In this problem

a=$1,200

r=1.1

n=18

substitute

`\begin{gathered} S=(1,200(1-1.1^((18))))/(1-1.1) \\ S=\$54,719.01 \end{gathered}`

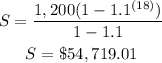
answer Part C is $54,719.01