Question

10. Provide the reasons for the following proof:

Given: Line WX =~ Line XY, Line XZ bisects Angle WXY
Prove: Triangle WXZ =~ Triangle YXZ

The last answer choice is symmetric property of =~;SAS

Am I correct?

Answer 1

The reason for third statement is reflexive property and the reason for fourth statement is SAS.

Explanation:

Given information:
`WX\cong XY` and XZ bisects angle WXY.

To prove:
`\trinagle WXZ\cong \triangle YXZ`

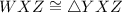
Statement Reason

`WX\cong XY` (Given)

`\angle WXZ\cong \angle YXC` (XZ bisects angle WXY)

`XZ\cong ZX` (Reflexive property)

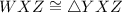`\trinagle WXZ\cong \triangle YXZ` SAS

Reflexive property states that a line is congruent to itself.

SAS postulate states that two triangle are congruent If two sides and the included angle of one triangle are equal to two sides and included angle of another triangle.

Therefore the reason for third statement is reflexive property and the reason for fourth statement is SAS.

Answer 2

Given that Line WX is congruent to Line XY and Line XZ bisects Angle WXY.

We prove that triangle WXZ is congruent to triangle YXZ as follows:


`\begin{tabular} Statement&Reason\\[1ex] \overline{WX}\cong\overline{XY},\ \overline{XZ}\ bisects\ \angle WXY&Given\\ \angle WXY\cong\angle YXZ & Deifinition of an angle bisector\\ \overline{XZ}\cong\overline{ZX}&Refrexive Property of \cong\\ \triangle WXZ\cong\triangle YXZ&SAS \end{tabular}`