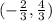ANSWER
The centroid of triangle JKL is
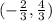
Step-by-step explanation
The centroid is the point where the medians of the triangle will intersect.
The median is the line from one vertex of the triangle and goes through the midpoint of the opposite side of the triangle.
So we find any two midpoints as follows;
Midpoint of K(2,4) and L(0,-2).
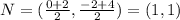
Midpoint of J(-4,2) and K(2,4),
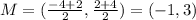
We now find the equation of the line that passes through J(-4,2) and N(1,1)
The slope is
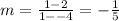
We use the point-slope form formula,
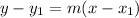
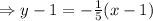
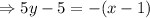

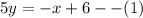
We also find the equation through L(0,-2) and M(-1,3)
The slope is
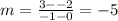
We use the point-slope form formula,
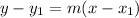

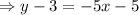

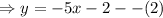
We substitute equation (2) in equation (1),
This gives us,
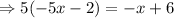
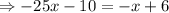
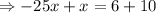
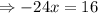
We divide both sides by -24 to obtain,
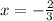
We substitute
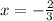
in to equation (2) to obtain,
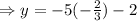
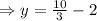
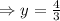
Therefore the centroid is