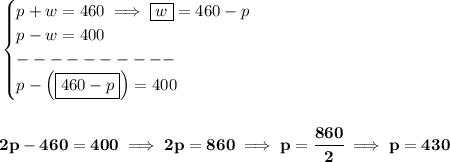Question

A plane traveled 460 mph with the wind and 400 mph against the wind. Find the speed of the plane in still air and the speed of the wind.

Answer 1

let's say the speed of the plane is "p", and the speed of the wind is "w".

when the plane goes with the wind, is not really going "p" mph fast, is really going "p + w" mph fast, because the wind is adding speed to it.

likewise when the plane is going against the wind, is also not going "p" fast is going "p - w" mph fast. Therefore,


`\bf \begin{cases} p+w=460\implies \boxed{w}=460-p \\ p-w=400\\ ----------\\ p-\left( \boxed{460 - p} \right)=400 \end{cases} \\\\\\ 2p-460=400\implies 2p=860\implies p=\cfrac{860}{2}\implies p=430`