Question

A ball is thrown from an initial height of 6 feet with an initial upward velocity of 17 ft's. The ball's height h (in feet) after t seconds is given by the following.h=6+17t-16t^2Find all values of t for which the ball's height is 10 feet.Round your answer(s) to the nearest hundredth(If there is more than one answer, use the "or" button.)

Answer 1

Given:

The height (h) of the ball after t seconds is given by the relationship:

`h=6+17t-16t^2`

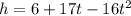
Solution

We are required to find the values of t for which the ball's height is 10 feet.

We set h = 10 feet and then solve the resulting equation.

`10=6+17t-16t^2`

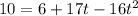
We can then solve the equation:

`\begin{gathered} -16t^2\text{ + 17t + 6 - 10 = 0} \\ -16t^2\text{ + 17t - 4 = 0} \\ \text{Divide through by -1} \\ 16t^2\text{ - 17t + 4 = 0} \\ U\sin g\text{ quadratic formular,} \\ x\text{ = }\frac{-b\text{ }\pm\sqrt[]{b^2-4ac}}{2a} \\ we\text{ have,} \\ t\text{ = 0.71s or 0.35s (nearest hundredth)} \end{gathered}`

Answer: 0.71 sec or 0.35 sec