Answer:
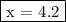
Step-by-step explanation:
Using the pythangorean theorem
[a² + b² = c²], we can solve enough sides to find the proportion because both triangles are right triangles or have a 90° right angle.
Given the lengths of side a and c (the hypotenuse), we can rearrange the pythangorean theorem to solve for b.
a² + b² = c² → a² – a² + b² = c² – a² →
b² = c² – a² → b = √(c² – a²).
We are also given the hypotenuse or the side c of both triangle which are 5 to 7, which is the proportion between the triangles. We also know they are similar because they have one congruent angle and a right angle, thus the third angle must be the same. Therefore the bigger triangle has sides that are 7/5 longer than the smaller triangle. So b of the smaller triangle = √(5² – 4²) = √(25 – 16) = √9 = 3.
Now we know the sides of the smaller triangle:
a = 3
b = 4
c = 5
______
Now the only thing we have to do is match the corresponding sides, and multiply by 7/5.
This means that the sides of the bigger triangle are:
a = 3 × 7/5 = 21/5 = 4.2
b = 4 × 7/5 = 28/5 = 5.6
c = 5 × 7/5 = 7
______________
Each angle corresponds to the side opposite or across from it. Since side a is across from the congruent pair of angles we are given, the a of the bigger triangle must be x which means that x = a = 4.2.