Question

Graph the system of linear inequalities and shade in the solution set. If there are no solutions, graph the corresponding lines and do not shade in any region - 2x + y > 2 Y greater and equal to - 2

Answer 1

To solve a system of linear inequalities, we preccend similarly to when we have a system of equations.

We have the two inequalities:

`\begin{cases}-2x+y\ge2 \\ y\ge-2\end{cases}`

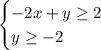
Let's solve the first inequality for y:

`\begin{gathered} -2x+y\ge2 \\ y\ge2+2x \end{gathered}`

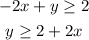
Now, we can graph both equations. To graph the first inequality, we can draw the line y = 2 +2x and shade all the region above that line (because we have y greater or equal than")

To graph a line, we just need to find two points and connect them:

Let's see the value of y for x = 0 and x = 1:

`\begin{gathered} y=2+2\cdot0\Rightarrow y=2 \\ y=2+2\cdot1\Rightarrow y=4 \end{gathered}`

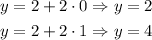
Now we have the points (0, 2) and (1, 4). We can locate them in the cartesian plane and connect them wiht a line:

And since is an inequality, the solutions are the points above the line.

Then we have:

For the second inequality, the border line is y = -2. Is a line parallel to x-axis that passes through y = -2:

And since the inequality is all y bigger or equal to -2, we shade the section above the line:

If we draw the two regions in the same graph we get:

The part the two shades intersect are all the solutions to the system of inequalities.