You need to firstly show that
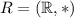
is a group, which requires that
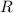
is closed under

, that

is associative,, that
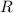
has an identity element, and that every element in
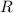
has a corresponding inverse in
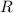
. Then for
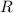
to be abelian, you also need to show that

is commutative.
But suppose
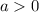
and

, where
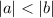
. For instance, take

and
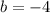
. Then
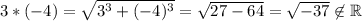
which means
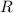
is not closed under

and is therefore not a group, and certainly not an abelian one.