Question

If you attach a 50.0 g mass to the spring whose data are shown in the graph, what will be the period of it's oscillations?

A. 8.9 s
B. 0.28 s
C. 1.4 s
D. 13 s

Answer 1

i thinks it's c. 14 but im not sure 

Answer 2

B. 0.28 s

Step-by-step explanation:

From the graph, we can calculate the spring constant of the spring, which is equal to the slope of the force-displacement graph. Taking two random points on the graph, we find:

`k=(\Delta F)/(\Delta x)=(2.00 N -1.00 N)/(0.08 m-0.04 m)=25 N/m`

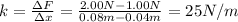
The period of oscillations is given by:

`T=2\pi \sqrt{(m)/(k)}`

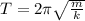
where m=50.0 g=0.05 kg is the mass on the spring. Substituting numbers, we find

`T=2\pi \sqrt{(0.05 kg)/(25 N/m)}=0.28 s`