Question

Find the coordinates of the circumcenter of triangle ABC with vertices A(0,0) B(3,0) and C(3,2)

A (0,0)
B (1.5,1)
C (2.5,1.5)
D (6,2)

Answer 1

B. (1.5,1)

Explanation:

Answer 2

The circumcenter of the given triangle is given by the iintersection of the perpendicular bisectors of the given triangle.

The midpoint of side AB with points A(0, 0) and B(3, 0) is given by


`\left( (0+3)/(2),\ (0+0)/(2) \right)=\left( (3)/(2),\ (0)/(2) \right) \\ \\ =(1.5,0)`

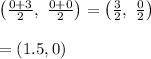
The equation of the line passing through A(0, 0) and B(3, 0) is given by


`(y)/(x) = (0)/(3) =0 \\ \\ \Rightarrow y=0`

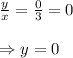
The equation of the line perpendicular to line y = 0 and passing through the point (1.5, 0) is given by x = 1.5

The midpoint of side BC with points B(3, 0) and C(3, 2) is given by


`\left( (3+3)/(2),\ (0+2)/(2) \right)=\left( (6)/(2),\ (2)/(2) \right) \\ \\ =(3, 1)`

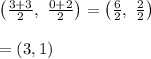
The equation of the line passing through B(3, 0) and C(3, 2) is given by


`(y)/(x-3) = (2-0)/(3-3) =(2)/(0)\\ \\ 2(x-3)=0\\ \\ x-3=0 \\ \\ \Rightarrow x=3`

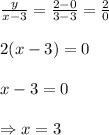
The equation of the line perpendicular to line x = 3 and passing through the point (3, 1) is given by y = 1

The point of intersection of the line x = 1.5 and the line y = 1 is (1.5, 1)

Therefore, the coordinates of the circumcenter of triangle ABC with vertices A(0,0) B(3,0) and C(3,2) is (1.5, 1)