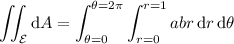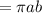Question

Use double integrals to calculate the area inside the ellipse whose semiaxes have length a and b

Answer 1

The general equation of an ellipse centered at the origin with its semiaxes coinciding with the coordinate axes is given by


`(x^2)/(a^2)+(y^2)/(b^2)=1`

Substituting
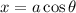
`x=a\cos\theta` and
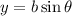
`y=b\sin\theta` into the above equation gives the Pythagorean identity, so we can use polar coordinates quite nicely to our advantage.

If
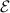
`\mathcal E` is the ellipse with the equation above, the area is given by the double integral


`\displaystyle\iint_(\mathcal E)\mathrm dA=\iint_((x/a)^2+(y/b)^2\le1)\mathrm dx\,\mathrm dy`

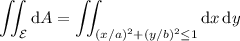
Let
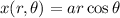
`x(r,\theta)=ar\cos\theta` and
`y(r,\theta)=br\sin\theta`, so that the Jacobian matrix is


`\mathbf J=\begin{bmatrix}x_r&x_\theta\\y_r&y_\theta\end{bmatrix}=\begin{bmatrix}a\cos t&-ar\sin t\\b\sin t&br\cos t\end{bmatrix}`

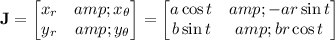
and the magnitude of its determinant is
`|\det\mathbf J|=|abr|=abr`

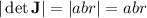
since in polar coordinates we use the convention that
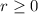
`r\ge0`, and
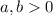
`a,b>0` because they are lengths.

Now, the area is given by


`\displaystyle\iint_(\mathcal E)\mathrm dA=\int_(\theta=0)^(\theta=2\pi)\int_(r=0)^(r=1)abr\,\mathrm dr\,\mathrm d\theta`

`=\displaystyle2\pi a b\int_(r=0)^(r=1)r\,\mathrm dr`

`=\pi a b`