The augmented matrix which represents the system of equations is
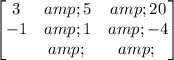
Now, lets solve the system by reducing this matrix to row echelon form.
By dividing the first row by 3, we have
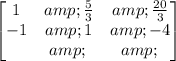
Now, by adding the rows, we get
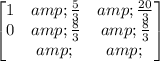
By multiplying the second row by 8/3, we have

and by multiplying the second row by -5/3, we get
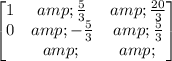
and by adding the second row to the first rwo, we obtain

therefore, the final result is
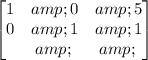
This means that the solution is x=5 and y=1. Then, the solution is (5,1)