Question

(Picture Provided) A line passes through the points

(6,5)
and
(2,2)

Select Yes or No to tell whether each equation describes this line.

Answer 1

only the top one is yes other 3 are no

Answer 2

Given that : A line passes through the points (6,5) and (2,2).

We need to select the forms of linear equations given:

First we need to find the slope between (6,5) and (2,2) points first.

`\mathrm{Slope\:between\:two\:points}:\quad \mathrm{Slope}=(y_2-y_1)/(x_2-x_1)`

`m=(2-5)/(2-6) =(3)/(4)`

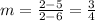
So, we got slope of the equation: 3/4.

Taking point (6,5) and slope 3/4.

Using point -slope form y-y1 =m(x-x1), we get

y-5 = 3/4 (x-6).

Taking point (2,2) and slope 3/4, we get

y-2 = 3/4 (x-2).

Therefore, correct options are First and Fourth options.