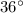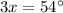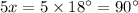Answer:
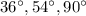
Explanation:
Given
Triangle is right-angled
The ratio of smallest and largest angle is 3:5
let the angles be 3x and 5x
In a right-angled triangle, the largest angle is
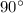
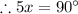
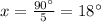
3x is the smallest angle. Its complementary angle is
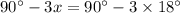
Complementary angle is