The probability excercise assumes experiments that are independent without repetition. In this case each experiment to get a baby will have a success if it were a girl with probability 0.5, then you have a binomial distribution with n=23 and p=0.5. Then
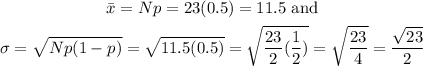
The range rule of thumb defines the range about four times the standard deviation, that in this case the range is the difference between the significantly high and low results. It means that
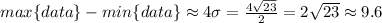
Then also for the range rule you have that the values around the mean are given by
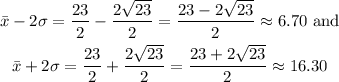
The range rule is really usefull because it indicates that most values would be in the area that is covered by four standard deviation, in other words two times the standard deviation after the mean and two times the standar deviation before the mean you will get the results that are significantly high and significantly low respectively.
Then values of 6.7 girls or FEWER are significantly low and the values of 16.30 girls or GREATER are significantly high.