Question

Find the coordinates of K'after a 90°

clockwise rotation about the origin.
K(-2,3)
A. (2,-3)
B. (-3,-2)
C. (3,2)
D. (-2,-3)

Answer 1

Given:

The coordinates of the given point are K(-2,3).

To find:

The coordinates of K' after a 90° clockwise rotation about the origin.

Solution:

We know that, if a figure rotated 90° clockwise about the origin, then the rule of rotation is defined as

`(x,y)\to (y,-x)`

Using this rule, we get

`K(-2,3)\to K'(3,-(-2))`

`K(-2,3)\to K'(3,2)`

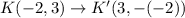
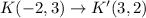
The coordinates of point K' are (3,2).

Therefore, the correct option is C.