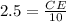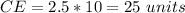Question

In the figure, AB is parallel to CD. If the area of triangle CED is 425, the area of triangle BEA is 68, and BE = 10, find CE.

Answer 1

62.5 units would be the answer bc 425/68=6.25 and 6.25*10 =62.5

Answer 2

`CE=25\ units`

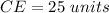
Explanation:

we know that

If AB is parallel to CD. then triangles CED and BEA are similar

If two figures are similar, the ratio of its areas is equal to the scale factor squared

Let

z------> the scale factor

x-----> the area of triangle CED

y-----> the area of triangle BEA

so

`z^(2)=(x)/(y)`

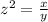
we have

`x=425\ units^(2)`

`y=68\ units^(2)`

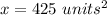
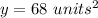
substitute and solve for z

`z^(2)=(425)/(68)`

`z^(2)=6.25`

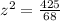
`z=2.5` ------> the scale factor

Remember that

If two figures are similar, the ratio of its corresponding sides is equal to the scale factor

so

`z=(CE)/(BE)`

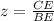
we have

`z=2.5`

`BE=10\ units`

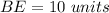
substitute and solve for CE

`2.5=(CE)/(10)`

`CE=2.5*10=25\ units`