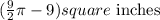Question

Find the area of one segment formed by a square with sides of 6” inscribed in a circle

Answer 1

Given that the square has sides 6'' and is inscribed in a circle, the figure is as shown below:

Step 1: Evaluate the length of the diagonal BD.

Thus, from the Pythagorean theorem,

`\begin{gathered} (hypotenuse)^2=(opposite)^2+(adjacent)^2 \\ where \\ BD\Rightarrow hypotenuse \\ \end{gathered}`

Thus,

`\begin{gathered} |BD|^2=6^2+6^2 \\ =36+36 \\ \Rightarrow|BD|^2=72 \\ Take\text{ the square root of both sides,} \\ √(|BD)|^2=\sqrt{{}72} \\ \Rightarrow|BD|=6√(2) \end{gathered}`

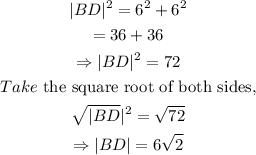
Step 2: Evaluate the radius of the circle.

The radius of the circle is exactly half the length of the diagonal.

Thus,

`\begin{gathered} radius\text{ of the circle = }(|BD|)/(2) \\ =(6√(2))/(2) \\ \Rightarrow radius\text{ of the circle = 3}√(2) \end{gathered}`

Step 3: Evaluate the area of the circle.

The area of the circle is expressed as

`\begin{gathered} Area\text{ of circle = }\pi r^2 \\ where \\ r=3√(2) \\ thus, \\ area\text{ = }\pi*(3√(2))^2 \\ \Rightarrow area\text{ = 18}\pi\text{ square inches} \end{gathered}`

Step 4: Evaluate the area of the square.

The area of a square is evaluated as

`\begin{gathered} area\text{ of square = L}^2 \\ where \\ L\text{ is the length of a side of the square} \\ thus,\text{ when L=6} \\ we\text{ have} \\ area\text{ of square = 6}*6 \\ \Rightarrow area=36\text{ square inches} \end{gathered}`

Step 5: Subtract the area of the square from the area of the circle.

Thus,

`\begin{gathered} (18\pi-36)\text{ square inches} \\ \end{gathered}`

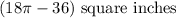
Step 6: Divide the obtained area by the number of segments.

The obtained area is the total area of the segments in the above figure.

Thus, to obtain the area of one segment, we divide by the total number of segments.

Thus,

`\begin{gathered} \frac{(18\pi-36)}{number\text{ of segments}}=((18\pi-36))/(4) \\ \Rightarrow((9)/(2)\pi-9)square\text{ inches} \end{gathered}`

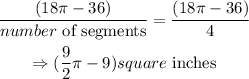
Hence, the area of one segment formed by the square is

`\begin{equation*} ((9)/(2)\pi-9)square\text{ inches} \end{equation*}`