Question

What is the slope of the line graphed on the coordinate plane? A graph with a line running through coordinates (0, 6) and coordinates (1, -2)

Answer 1

`m=-8`

Explanation:

We have been given coordinates of two points on a line and we are asked to find the slope of the line running through the given points.

We will use slope formula to solve our given problem.

`m=(y_2-y_1)/(x_2-x_1)`, where,

`m=\text{Slope of line}`,

`y_2-y_1=\text{Difference between two y-coordinates}`,

`x_2-x_1=\text{Difference between two x-coordinates of same y-coordinates}`.

Let point
`(0,6)=(x_1,y_1)` and point
`(1,-2)=(x_2,y_2)`.

Upon substituting coordinates of our given points in slope formula we will get,

`m=(-2-6)/(1-0)`

`m=(-8)/(1)`

`m=-8`

Therefore, the slope of line passing through our given points is
`-8`.

Answer 2

The slope of the line is -8

Explanation

To find the slope of our line we are going to use the slope formula:

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

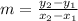
where

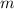`m` is the slope of the line

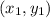`(x_(1),y_(1))` are coordinates of the first point

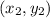`(x_(2),y_(2))` are the coordinates of the second point

We know that the first point on our graph is (0, 6), so
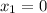`x_(1)=0` and
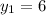`y_(1)=6`. We also know that the second point is (1, -2), so
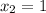`x_(2)=1` and
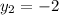`y_(2)=-2`. Let's replace those values in our formula:

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

`m=(-2-6)/(1-0)`

`m=(-8)/(1)`

`m=-8`

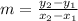
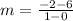
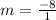
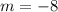
We can conclude that the slope of the line passing through the points (0, 6) and (1, -2) is -8.