Question

A beach has two floating docks. One is 650 meters east of the lifeguard stand. The other is 60° southeast and 750 meters from the lifeguard stand. The distance between the docks is____ Rounded to the nearest meter.

A) 589
B) 705
C) 792
D) 861

Answer 1

using the cosine law

Cos60 = (650^2 +750^2 - x^2)/(2*650*750) x is the distance between them

(2*650*750)cos60 = 650^2 + 750^2 - x^2

x^2 = 650^2 +750^2 - (2*650*750)cos60 =497500

x = √497500 = 705 meters approx.

Answer 2

Answer: Option 'B' is correct.

Explanation:

Since we have given that

Distance between the lifeguard and the first beach = 650 meters

Distance between the lifeguard and the second beach = 750 meters

As shown in the figure below :

We will use "Cosine formula ":

We need to find the distance between the docks is given by

`\cos A=(b^2+c^2-a^2)/(2bc)\\\\\cos 60\textdegree=(650^2+750^2-x^2)/(2* 650* 750)\\\\(1)/(2)=(422500+562500-x^2)/(975000)\\\\(975000)/(2)=985000-x^2\\\\487500-985000=-x^2\\\\497500=x^2\\\\√(497500)=x\\\\705.33=x\\\\x=705\ meters`

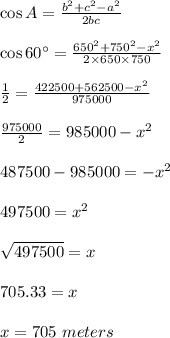
Hence, Option 'B' is correct.