Question

I have this homework question that's been confusing me: an object is fired upwards with an initial velocity of 112 ft per second. It's height, in feet above the ground, as a function of time, in seconds since it was fired is given by the equation h(t) = -16t^2 + 112t At what height was the object fired? Algebraically find the time that the rocket reaches its greatest height and the maximum height.

Answer 1

Substitute 0 for t in the height equation to determine the height from where object was fired.

`\begin{gathered} h(0)=-16(0)^2+112\cdot0 \\ =0+0 \\ =0 \end{gathered}`

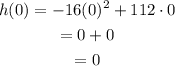
So object is fired from the ground or at a height of 0 feet from the ground.

At maximum height the velocity of the object is 0.

Differentiate the height equation to determine the veleocity equation for the object.

`\begin{gathered} (d)/(dt)h(t)=(d)/(dt)(-16t^2+112t) \\ =(d)/(dt)(-16t^2)+(d)/(dt)(112t) \\ =-32t+112 \end{gathered}`

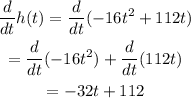
Equate the derivative to 0 to determine the time at which object reached the maximum height.

`\begin{gathered} -32t+112=0 \\ t=-(112)/(-32) \\ =3.5 \end{gathered}`

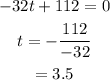
Substitute 3.5 for t in the height equation to determine the maximum height at which rocket reached.

`\begin{gathered} h(3.5)=-16(3.5)^2+112\cdot3.5 \\ =-196+392 \\ =196 \end{gathered}`

Plot the height equation on the graph.

In graph it can be observed that curce attain the peak at 3.5 at maximum value is 196.

So maximum height attain by the rocket is 196 feet.