Question

Line segment CD begins at (-1, 2) and ends at (6, 2). The segment is translated 4 units down and 2 units left. What is the length of line segment C'D'? (A.) 2 units (B.) 4 units (C.) 5 units (D.)7 units

Answer 1

The answer is B, with a translation the segement size doesn’t change.

Answer 2

Option D.

Explanation:

It is given that line segment CD begins at (-1, 2) and ends at (6, 2).

The segment is translated 4 units down and 2 units left.

We know that translation is a rigid transformation. It means, after translation the size and shape of figure remains same.

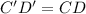`C'D'=CD` ... (i)

Using distance formula, the length of CD is

`CD=√((x_2-x_1)^2+(y_2-y_1)^2)`

`CD=√((6-(-1))^2+(2-2)^2)`

`CD=√((6+1)^2+(0)^2)`

`CD=√(49)`

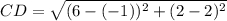
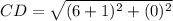
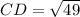
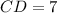`CD=7` ... (ii)

Using (i) and (ii), we get

`C'D'=CD=7\text{ units}`

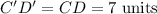
Therefore, the correct option is D.