Answer:
146°
Explanation:
You're given an angle, where the vertex is the center of a circle. This makes the angle's rays two radii of the circle. You are then given the length of 1/360th of the circle, which is .51cm, which is also 1 degree of the circle.
If the arc of the circle between the angle's rays is 74.46 cm. It becomes a division problem:
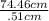
After the division, the fraction simplifies to 146, which is 146 segments of .51cm in the arc, and since .51cm is equivalent to 1 degree of the circle, the arc is 146 degrees.