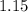Question

In the exponential growth function y = 235(1.15)^x, what is the

1. growth factor? _____________
2. percent increase? _____________

Answer 1

Growth Factor: 1.15
The y-values multiply by 1.15 every unit you increase x by.
Percentage Increase: 15%
If we are multiplying y-values by 1.15 for every unit of x, we are finding 115% (= 1.15 × 100) of the previous value of y;
100% is equivalent to the previous value, so effectively, we are just adding to the previous value 15% of itself to get the next value.
E.g. If x = 1, then:
y = 235(1.15)¹
= 270.25;
The y-value when x = 2 is just:
270.25 + (15% of 270.25)
= 270.25 + (270.25 × (15/100))
= 270.25 + (40.5375)
= 310.7875
The easier way to write and represent this for any value of x is:
y = 235(1.15)ˣ

Answer 2

Answer: 1. growth factor = 1.15

2. percent increase = 15%

Explanation:

The exponential growth function is given by :-

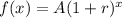`f(x)=A(1+r)^x`, where A is the initial amount , r is the rate of growth and x is the time period.

Here, The growth factor=
`1+r`

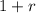
The given exponential function :
`f(x)=235(1.15)^x=235(1+0.15)^x`

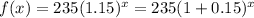
When we compare to the general exponential function , we get

The rate of growth :
`0.15`

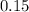
Thus, the percent increase = 15%

The growth factor=
`1.15`