Question

In a random sample of 19 patients at a hospital's emergency room, the mean waiting time before seeing a doctor was 23 minutes and the sample standard deviation was 11 minutes. a) construct a 95% confidence interval using the Z or Interval b) state which interval was used - Z or T Interval

Answer 1

We have to calculate a 95% confidence interval for the mean.

The population standard deviation is not known, so we have to estimate it from the sample standard deviation and use a t-students distribution to calculate the critical value.

The sample mean is M=23.

The sample size is N=19.

When σ is not known, s divided by the square root of N is used as an estimate of σM:

`s_M=(s)/(√(N))=(11)/(√(19))=(11)/(4.359)=2.524`

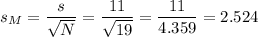
The degrees of freedom for this sample size are:

`df=n-1=19-1=18`

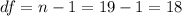
The t-value for a 95% confidence interval and 18 degrees of freedom is t=2.101.

The margin of error (MOE) can be calculated as:

`MOE=t\cdot s_M=2.101\cdot2.524=5.302`

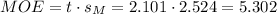
Then, the lower and upper bounds of the confidence interval are:

`\begin{gathered} LL=M-t\cdot s_M=23-5.302=17.698 \\ UL=M+t\cdot s_M=23+5.302=28.302 \end{gathered}`

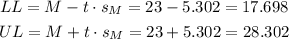
The 95% confidence interval for the mean is (17.698, 28.302).

The interval is a T-interval, as the sampleis small and we don't know the standard deviation of the population.