Answer:
The point slope form of required line is
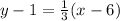 .
.
Explanation:
It is given the the required line passes through the point (6,1) and perpendicular to a line with a slope of -3.
The product of slopes of two perpendicular lines is -1.
Let the slope of required line be m.
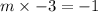
Divide both sides by -3.
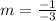
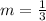
The slope of required line is 1/3.
If a line passes through the point
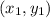 with sloe m, then the point slope form of the line is
with sloe m, then the point slope form of the line is
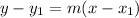
The point slope form of required line is
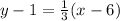
Therefore the point slope form of required line is
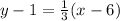 .
.