Question

Let (7, 24) be a point on a graph. If you draw a segment from the origin to the point, determine the angle that is formed between the segment and the x-axis. Round to the nearest tenth

Answer 1

Answer and Step-by-step explanation:

The angle that is formed is an acute angle, which means it has to be less than 90 degrees.

The length from the point to the origin (hypotenuse) is 25 (obtained by using the distance formula).

The height of the triangle is 24, since it goes up 24 units, and the base of the triangle is 7, since it goes right 7 units.

↑

| [.] (7, 24)

| /|

| / |

| / |

| 25 / |

| / | 24

| / |

| / |

|/_______|_______→

| 7

|

↓

[Triangle is not to scale]

We can use any trig function to find the angle.

Let's use tangent.

tan(θ) =
`(24)/(7)`

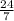
θ =
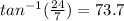`tan^(-1) ((24)/(7) ) = 73.7` is the angle.

#teamtrees #WAP (Water And Plant)