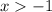Question

1+ x>2(-2-5x)+6xSolve for x

Answer 1

x > -1

Step-by-step explanation:

We have:

`1+x>2\cdot(-2-5x)+6x`

In order to solve for x, we have to isolate x.

To do this, we begin solving each side:

`\begin{gathered} 1+x>-4-10x+6x \\ 1+x>-4-4x \end{gathered}`

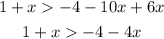
To the inequality keep being true, we have to do the same thing with both sides of the equation.

So, let's sum -1 in both sides:

`\begin{gathered} 1+x-1>-4-4x-1 \\ x>-5-4x \end{gathered}`

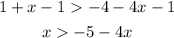
Now let's sum +4x on both sides:

`\begin{gathered} x+4x>-5-4x+4x \\ 5x>-5 \end{gathered}`

Finally, let's divide both sides by 5:

`\begin{gathered} (5x)/(5)>-(5)/(5) \\ x>-1 \end{gathered}`

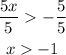
Answer:

`x>-1`