Question

What is the approximate value of sin C?

Answer 1

(D) 0.36

Explanation:

Given: It is given that in ΔABC, which is right angled at A has AC=13, AB=5 and BC=13.93.

To find: The value of sinC.

Solution: It is given that in ΔABC, which is right angled at A has AC=13, AB=5 and BC=13.93. thus, using the trigonometry that is:

`sinC=(Perpendicular)/(Hypotenuse)`

`sinC=(AB)/(BC)`

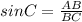
Substituting the given values, we have

`sinC=(5)/(13.93)`

`sinC=0.36`

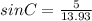
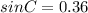
which is the required value.

Hence, option D is correct.

Answer 2

Find the approximate value of sin C .

To proof

By using the trignometric identity

`sin\theta = (Perpendicular)/(Hypotenuse)`

Now as given in the diagram

`sin C = (AB)/(BC)`

as in the diagram

AB = 5 units

BC = 13.93 units

Now put it in the identity

`sinC = (5)/(13.93)`

solving

sinC = 0.36° (approx)

Option (D) is correct

Hence proved