Question

What is normal, when it comes to people's body temperature? a random sample of 130 human body temperatures, provided by allen shoemaker in the journal of statistical education, had a mean of 98.25◦ and a standard deviation of 0.73◦ . (a) does the data indicate that the average body temperature for healthy humans is different from 98.6◦ , the usual average temperature cited by physicians and others? test using α = 0.01. (b) what is the p-value?

Answer 1

The two-sided alternative hypothesis is appropriate in this case, the reason being we are asked "does the data indicate that the average body temperature for healthy humans is different from 98.6◦........?".
The test statistic is:

`Z= \frac{\bar{X}-\mu}{ (s)/( √(n) ) }} =-5.47`
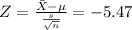
Using an inverse normal table, and halving
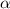
`\alpha` for a two-tailed test, we look up
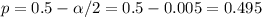
`p=0.5-\alpha/2=0.5-0.005=0.495` and find the critical value to be Z = 2.5758.
Comparing the test statistic Z = -5.47 with the rejection region Z < -2.5758 and Z > 2.5758. we find the test statistic lies in the rejection region. Therefore the evidence does not indicate that the average body temperature for healthy humans is different from 98.6◦.