Question

Solve this math problem: a bag of coins contains nickels, dimes, and quarters. there are a total of 21 coins in the bag, and the total amount of money in the bag is $3.00. there is one more dime than there are nickels. how many dimes, nickels, and quarters are in the bag?

Answer 1

Solve this math problem: a bag of coins contains nickels, dimes, and quarters. there are a total of 21 coins in the bag, and the total amount of money in the bag is $3.00. there is one more dime than there are nickels. how many dimes, nickels, and quarters are in the bag?

Answer 2

Answer: There are 6 nickels, 7 dimes and 8 quarters in the bag.

Step-by-step explanation: Given that the bag contains coins of nickels, dimes and quarters. There are total 21 coins in the bag and number of dimes is one more than the number of nickels.

Let 'x' be the number of nickels in the bag.

So, number of dimes will be (x+1) and number of quarters will be (21-x-x-1) i.e., (20-2x).

Since there are total $3.00 amount of money in the bag, so

`0.05x+0.10(x+1)+0.25(20-2x)=3\\\\\Rightarrow(x)/(20)+(x+1)/(10)+(20-2x)/(4)=3\\\\\Rightarrow (x+2x+2+100-10x)/(20)=3\\\\\Rightarrow -7x+102=60\\\\\Rightarrow 7x=42\\\\\Rightarrow x=6.`

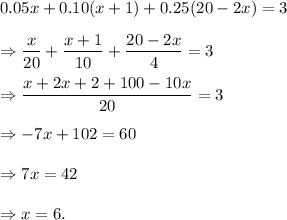
Therefore, number of nickels = 6, number of dimes = 6+1 = 7 and number of quarters = 20-2×6 = 8.

Thus, there are 6 nickels, 7 dimes and 8 quarters in the bag.