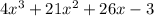I assume for this problem you are looking for the derivative?
If so. I hope this helps!
Apply the sum difference rule > d/dx(x^4) + d/dx(7^3) + d/dx(13x^2) - d/dx(3x) - d/dx(18)
For d/dx(x^4), d/dx(7^3), d/dx(13x^2), d/dx(3x), and d/dx(18). We want to take the constant out and the apply the power rule of
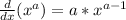
d/dx(x^4) >
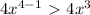
d/dx(7^3) >

d/dx(13x^2) >
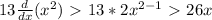
d/dx(3x) >
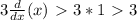
d/dx(18) >
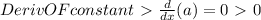
Now we can combine these all.
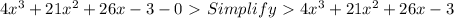
Therefore our final answer is