Question

Given a circle with measures of (C, d, and r) and a circle with measures of (C', d', and r'), what is C if d d' = 0.25 and C' = 16?

Answer 1

Mathematically, C’ = πd’

C’ =16, π = 22/7

∴ 16 = 22d’/7

16 x 7 = 22d’

d' = (16 x 7) / 22

d’ = 112/22

d’ = 5.09

d d’ = 0.25

d’ = 5.09

∴ d (5.09) = 0.25

d = 0.25/5.09

d = 0.05

Mathematically, C = πd

π = 22/7, d = 0.05

∴ C = 22/7 x 0.05

C = 0.16

Answer 2

C = 4

Explanation:

Given : Given a circle with measures of (C, d, and r) and a circle with measures of (C', d', and r').

and
`(d)/(d')=0.25`

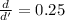
We have to find the value of C when C' = 16

Consider, The given circle with measures of (C, d, and r) and a circle with measures of (C', d', and r').

Since, Circumference of circle is directly proportional to the diameter of circle.

Thus, C ∝ d

and C' ∝ d'

Given
`(d)/(d')=0.25`

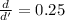
Thus,
`(C)/(C')=(d)/(d')=0.25`

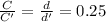
Simplify , we have,

C' = 16

`(C)/(16)=0.25`

Simplify, we get,

`C=0.25\time 16=4`

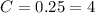
Thus, C = 4