Question

Directions: Find the area of each figure.(Hint: Round to the nearest tenth.)8 cmBlank 1:I

Answer 1

Let's suppose that the dashed line bisect the right angle and the figure is a square. Then, we can draw the following picture

then, we can draw the following right triangle

and we need to find x. This can be done as

`\cos 45=(x)/(8)`

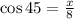
then x is equal to

`x=8\cos 45`

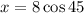
since cos 45 is

`\cos 45=\frac{1}{\sqrt[]{2}}`

then, x is given by

`x=\frac{8}{\sqrt[]{2}}`

We can note that the length of one side of our square is

`L=2x`

so, the one side is equal to

`\begin{gathered} L=2*\frac{8}{\sqrt[]{2}} \\ L=8\sqrt[]{2} \end{gathered}`

Since the area of a square is

`A=L^2`

the area of our figure is equal to

`\begin{gathered} A=(8\sqrt[]{2})^2 \\ A=64*2 \\ A=128 \end{gathered}`

That is, the area of the square is equal to 128 centimeters squared.