Question

Jasmine is going on a trip with her friend George. Theyboth decide to meetup at the location of the resort. Thedestination is at a 66 degree angle from Jasmine'shouse and a 53 degree angle from George's house. Ifthere is a 20 mile distance between George andJasmine respective houses, how far does George haveto drive to arrive at the resort? How far does Jasminehave to drive? For a visual aid see the attachment.*Triangle not drawn to scale.*

Answer 1

The total angles in a triangle are 180degrees.

Therefore,

`\begin{gathered} a+66^0+53^0=180^0 \\ a=180^0-(66^0+53^0)=61^0 \\ \therefore a=61^0 \end{gathered}`

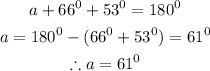
In order to solve for the distances of the other two sides, we will use the Sine rule formula.

which says,

`(\sin A)/(a)=(\sin B)/(b)=(\sin C)/(c)`

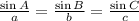
Where,

`\begin{gathered} \text{Jasmine's distance=b} \\ \text{George's distance=c} \\ R\text{esort}=a=20\text{miles} \\ A=61^0,B=66^0,C=53^0 \end{gathered}`

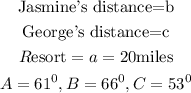
Solving for George's distance (c)

`\begin{gathered} (\sin61^0)/(20)=(\sin53^0)/(b) \\ c=\frac{s\text{in}53^0*20}{\sin61^0}=(0.79863551*20)/(0.8746197071)=18.26246318\approx18.26(nearest\text{ hundredths)} \\ c=18.26\text{miles} \end{gathered}`

Solving for Jasmine distance (b)

`\begin{gathered} (\sin A)/(a)=(\sin B)/(b) \\ (\sin61^0)/(20)=(\sin66^0)/(c) \\ b=(\sin66^0*20)/(\sin61^0)=(0.9135454576*20)/(0.8746197071)=20.8901183\approx20.89(nearest\text{ hundredths)} \\ \therefore b=20.89miles \end{gathered}`

Hence,

Jasmine will drive 20.89miles to arrive at the resort.

George will drive 18.26miles to arrive at the resort.