Question

The function f(x)=5(x−1) is shown on the coordinate plane. Select from the drop-down menus to correctly describe the end behavior of ​ f(x) ​. As x decreases without bound, the graph of f(x) . As x increases without bound, the graph of f(x) . An exponential curve graphed on a coordinate plane with horizontal x-axis ranging from negative 4 to 4 in increments of 1. The vertical y-axis ranges from negative 5 to 5 in increments of 1. The curve begins from the second quadrant. The curve increases through begin ordered pair 0 comma 0.2 end ordered pair. The curve passes through begin ordered pair 1 comma 1 end ordered pair and moves away from the positive y-axis.

Answer 1

Answer: As x decreases without bound the graph f(x) decreases, as x increases without bound the graph of f(x) increases.

Explanation:

Since, the given function is ,
`f(x) =5(x-1)`

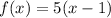
If
`x\rightarrow -\infty` then
`f(x)\rightarrow -\infty`

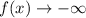
that is, we can say, as x decreases without bound the graph f(x) decreases.

While, If
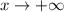`x\rightarrow +\infty` then
`f(x)\rightarrow +\infty`

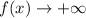
That is, we can say, as x increases without bound the graph f(x) increases.

thus, we can say first option represents the correct end behavior of f(x).