Question

The current of a river is 2 miles per hour. A boat travels to a point 8 miles upstream and bac in a total of 3 hours. What is the speed of the boat in still water?

Answer 1

speed in still water:x
speed upstream: (x-2)
speed downstream: (x+2)
time upstream: t
time downstream: (3-t)
distance=speed*time
8=(x-2)t
8=(x+2)(3-t)
solve the system of equation by replace t with x: t=8/(x-2)
8=(x+2)[3-8/(x-2)]
8=(x+2)[
`(3(x-2))/(x-2) - (8)/((x-2))` ]
8=(x+2)(
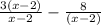
`(3x-14)/((x-2))`
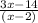
(x+2)(3x-14)=8(x-2)
3x^2-16x-12=0
x=6 or -2/3 (impossible because it is negative)
so the speed in still water is 6 miles per hour

check to see if it makes sense: upstream speed 4 miles per hours for a total of 8 miles, so that is 2 hours
downstream at speed 8 miles per hour for a distance of 8 miles, that's 1 hour
the total time is 3 hours

Answer 2

Boat Speed = S, Time upstream = t₁ ; Time downstream = t₂,
Distance upstream = Distance downstream = 8. We know that D = S.t

Speed of the boat upstream = S+2
Speed of the boat downstream = S-2; Then
Time travelled upstream = t₁ = Time travelled upstream = t₁ = 8/(S+2)
Time travelled downstream = t₂ = 8/(S-2)
t₁ + t₂ = 8/(S+2) + 8/(S-2) = 3 (t₁+t₂ = 3, given)

Solve 8/(S+2) + 8/(S-2) = 3→ 8(S-2)+8(S+2) = 3(S-2)(S+2)
→3S² -16S - 12 = 0 . Solving this quadratic equation gibes S = 6 and S =-2/3
So S = 6 mi/h the second S = -2/3 is EXTRANEOUS (unacceptable)